Directional Derivative & Gradient Vector
Notes from Paul’s Online Math
u: unit vector of direction
D: formula for computing directional derivatives
\[\vec{u} = \langle a, b \rangle \\ D_{\vec{u}} f (x, y) = f_x(x,y) a + f_y(x,y) b\]Example
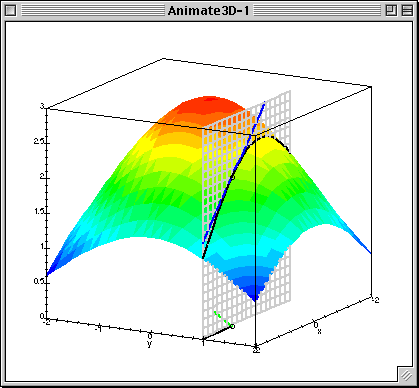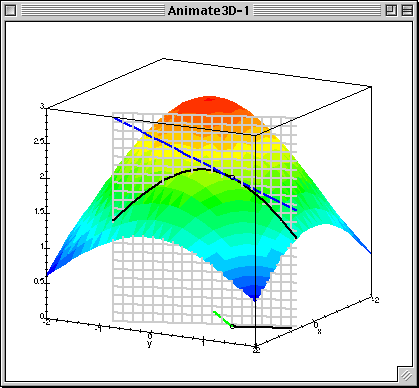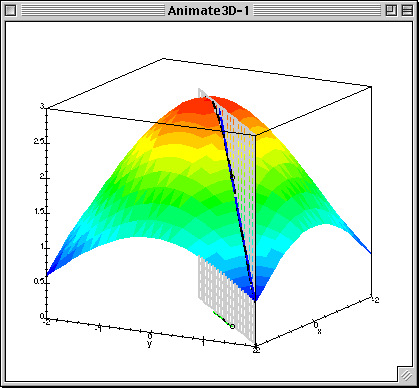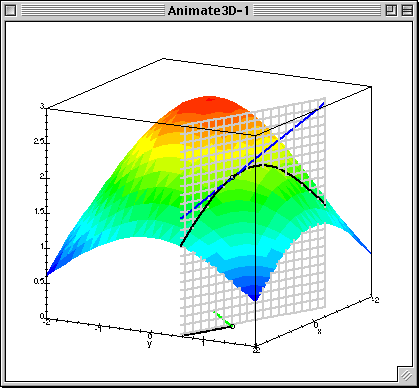
Find the directional derivatives of \(D_{\vec{u}} f(2,0)\) where \(f(x,y) = x e^{xy} + y\) and u is the unit vector in the direction of \(\theta = 2 \pi / 3\)
Mix Chain Rule, product rule, detail of using chain rule:
to solve the problem:
\[f_x = (x)' \times e^{xy} + x \times (e^{xy})' = e^{xy} + x \times y e^{xy} \\ f_y = x^2 e^{xy} + 1 \\ \vec{u} = ( \cos( \frac{2\pi}{3} ), \sin( \frac{2\pi}{3} ) ) = ( -\frac{1}{2}, \frac{\sqrt{3}}{2} ) \\ \begin{align} D_{\vec{u}} & = f_x \times a + f_y \times b \\ & = (e^{xy} + x y e^{xy}) \times -\frac{1}{2} + (x^2 e^{xy} + 1) \times \frac{\sqrt{3}}{2}, let x=2 \ \ y=0 \\ & = (1) \times -\frac{1}{2} + (4 + 1) \times \frac{\sqrt{3}}{2} \\ & = \frac{5\sqrt{3}-1}{2} \end{align}\]Gradient Vector of f
\[\begin{align} D_\vec{u} f (x,y,z) & = f_x(x,y,z) \ a + f_x(x,y,z) \ b + f_x(x,y,z) \ c \\ & = \langle f_x, f_y, f_z \rangle \cdot \langle a,b,c \rangle \\ & \\ \nabla f & = \langle f_x, f_y, f_z \rangle \\ & = f_x \vec{i} + f_y \vec{j} + f_z \vec{k} \\ D_\vec{u} f (x,y,z) & = \nabla f \cdot \vec{u} \end{align}\]The \(\vec{u}\) is the unit vector
Let \(\vec{x}\) be any number of variables x, y, z, …, or noted as \(x_1, x_2, x_3, \cdots\). We can also write the formula as:
\[D_\vec{u} f(\vec{x}) = \nabla f \cdot \vec{u}\]
Example
Find directional derivative \(D_{\vec{u}} f( \vec{x} )\), for \(f(x,y,z) = sin(yz) + ln(x^2)\) at \((1,1,\pi)\) in the direction of \(\vec{v} = \langle 1, 1, -1 \rangle\)
\[\begin{align} g(x) & = x^2 \\ h(z) & = ln(z) \\ f_x & = h'( g ) \cdot g' = \frac{2}{x} \\ f_y & = z \ \cos(yz) \\ f_z & = y \ \cos(yz) \\ \nabla f(x,y,z) & = \langle \ \ \frac{2}{x}, z \ \cos(yz), y \ \cos(yz) \ \ \rangle \\ \nabla f(1,1,\pi) & = \langle \ \ 2, \pi \ \cos(\pi), 1 \ \cos(\pi) \ \ \rangle \\ & = \langle \ \ 2, -\pi, -1 \ \ \rangle \\ \vec{v} & = \langle 1, 1, -1 \rangle \\ \vec{u} & = \frac{\vec{v}}{\Vert\vec{v}\Vert} = \langle \frac{1}{\sqrt{3}}, \frac{1}{\sqrt{3}}, \frac{-1}{\sqrt{3}} \rangle \\ D_{\vec{u}} f( 1,1,\pi ) & = \langle 2, -\pi, -1 \rangle \cdot \langle \frac{1}{\sqrt{3}}, \frac{1}{\sqrt{3}}, \frac{-1}{\sqrt{3}} \rangle \\ & = \frac{2}{\sqrt{3}} + \frac{-\pi}{\sqrt{3}} + \frac{1}{\sqrt{3}} \\ & = \frac{3 - \pi}{\sqrt{3}} \end{align}\]Theorem
- the maximum rate of change of function f
最大斜率:
The maximum value of \(D_{\vec{u}} f(\vec{x})\) is given by \(\Vert \nabla f(\vec{x}) \Vert\) and will occur in the direction given by \(\nabla f (\vec{x})\).
- 梯度 與 水平曲線
垂直
The gradient vector \(\nabla f(x_0, y_0)\) is orthogonal (or perpendicular) to the level curve f(x,y) = k at the point P(x0,y0). Likewise, the gradient vector \(\nabla f(x_0,y_0,z_0)\) is orthogonal to the level surface f(x,y,z)=k at the point (x0,y0,z0).